Number System: Dividing by Zero

Dividing by Zero

Don't divide by zero or this could happen! Just kidding.

In truth:

Dividing by Zero is undefined.

Dividing

To see why, let us look at what is meant by "division":

Division is splitting into equal parts or groups.
It is the result of "fair sharing".

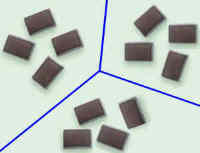
Example: there are 12 chocolates, and 3 friends want to share them, how do they divide the chocolates?

12 Chocolates		12 Chocolates Divided by 3

So they get 4 each: 12/3 = 4

Dividing by Zero

Now, let us try dividing the 12 chocolates among zero people, how much does each person get?
Does that question even make sense? No, of course it doesn't.
You can't share among zero people, and you can't divide by 0.

Another Good Reason

After dividing, can you multiply to get back again?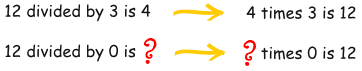

But multiplying by 0 gives 0, so that won't work.
Once again, dividing by zero gives us difficulties!

 

Imagine You Could Divide by Zero

Okay, let us imagine you can divide by zero, and see what happens.
That means that things like ¹/₀ and ⁰/₀ would behave like normal numbers.

Try Multiplying By Zero

So let us try using our new "numbers".
For example, we know that zero times any number is zero:

Example: 0×1 = 0, 0×2 = 0, etc

So that should also be true for ¹/₀:

0 × (¹/₀) = 0

But we could also rearrange it a little like this:

0 × (¹/₀) = (⁰/₀) × 1 = 1

(Careful! I am not saying this is correct! , we are assuming that you can divide by zero, so 0/0 should work the same as 5/5, which is 1).
Arrggh! If you multiply ¹/₀ by zero you could get 0 or 1.
In fact you can't have both possibilites, so we cannot define ¹/₀ to be a number.
So it is undefined.

So what is 0/0 ?

0/0 is like asking "how many 0s in 0?"
Are there no zeros in zero at all? Or perhaps there is exactly one zero in zero? Or many zeros?
So 0/0 is indeterminate (it could be any value).

In conclusion:

When you try to divide by zero, things stop making sense

That is all.

Posted in: Dividing By Zero, Number System